Page 113 - Ellingham, Mark, Mariusz Meszka, Primož Moravec, Enes Pasalic, 2014. 2014 PhD Summer School in Discrete Mathematics. Koper: University of Primorska Press. Famnit Lectures, 3.

mož Moravec: Some Topics in the Theory of Finite Groups 101

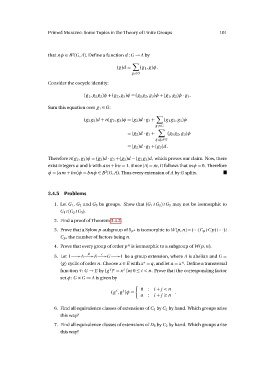
that n φ ∈ B 2(G , A). Define a function d : G → A by

(g )d = (g 1, g )φ.

g 1∈G

Consider the cocycle identity:

(g 1, g 2 g 3)φ + (g 2, g 3)φ = (g 1 g 2, g 3)φ + (g 1, g 2)φ · g 3.

Sum this equation over g 1 ∈ G :

(g 2 g 3)d + n (g 2, g 3)φ = (g 2)d · g 3 + (g 1 g 2, g 3)φ

g 1∈G

= (g 2)d · g 3 + (g 1 g 2, g 3)φ

g 1 g 2∈G

= (g 2)d · g 3 + (g 3)d .

Therefore n (g 2, g 3)φ = (g 2)d · g 3 + (g 3)d − (g 2 g 3)d , which proves our claim. Now, there
exist integers a and b with a m +b n = 1. Since |A| = m , it follows that m φ = 0. Therefore
φ = (a m + b n )φ = b n φ ∈ B 2(G , A). Thus every extension of A by G splits.

3.4.5 Problems

1. Let G1, G2 and G3 be groups. Show that (G1 G2) G3 may not be isomorphic to
G1 (G2 G3).

2. Find a proof of Theorem 3.4.2.

3. Prove that a Sylow p -subgroup of Spn is isomorphic to W (p, n ) = (· · · (Cp C p ) · · · )
Cp , the number of factors being n .

4. Prove that every group of order p n is isomorphic to a subgroup of W (p, n ).

5. Let 1 /A µ /E ε /G /1 be a group extension, where A is abelian and G =

〈g 〉 cyclic of order n . Choose x ∈ E with x ε = q , and let a = x n . Define a transversal

function τ: G → E by (g i )τ = x i for 0 ≤ i < n . Prove that the corresponding factor

set φ : G × G → A is given by

(g i , g j )φ = 0 : i+j a : i+j ≥n

6. Find all equivalence classes of extensions of C4 by C2 by hand. Which groups arise
this way?

7. Find all equivalence classes of extensions of D8 by C2 by hand. Which groups arise
this way?