Page 42 - Ellingham, Mark, Mariusz Meszka, Primož Moravec, Enes Pasalic, 2014. 2014 PhD Summer School in Discrete Mathematics. Koper: University of Primorska Press. Famnit Lectures, 3.
P. 42
1.8 Bouchet’s covering triangulations
Example: In K2 + C6 as shown, D has three components with vertex sets {p,q }, {u , w, y }
and {v, x , z }. Assuming all values of t1 are +1, we take t1 + α(q, p ) + α(u , w ) + α(v, x )
which has coefficient 2 for q and coefficient ±1 for everything else.
Note: As mentioned earlier, if all components of D are even order then works for any m ;
Bouchet gives other conditions that will guarantee this.
Folded coverings
If we want to extend Theorem above to even m , will be enough to do it for m = 2, then
can use induction for powers of 2 and combine with result for odd m . But it can be
shown that it is not always possible to get a generative 2-valuation.
Instead, need to use folded coverings [Bo82]. Original coverings have property that two
triangles containing given edge (x , i )(y , j ) correspond to the two distinct triangles
containing x y in G . But for folded covering, may have fold on edge (x , i )(y , j ): both
triangles containing this edge correspond to same original triangle (x y z ).
Theorem: Suppose Ψ is a triangulation of eulerian G . Then there is a triangular embed-
ding of G2) of the same orientability as Ψ, obtained by a folded covering.
Proof: Assign (x , t ) values as previously (±1 values at corners, alternating around each
vertex).
• For each x ∈ V (G ) let (x , −1) and (x , 1) be corresponding vertices in G(2).
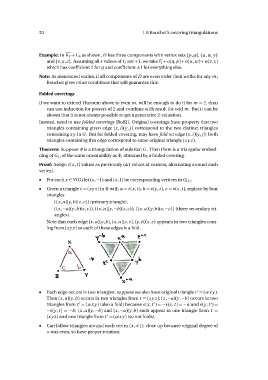
• Given a triangle t = (x y z ) in Ψ with a = (x , t ), b = (y , t ), c = (z , t ), replace by four
triangles
( (x , a )(y ,b )(z , c ) ) (primary triangle),
( (x , −a )(y ,b )(z , c ) ), ( (x , a )(y , −b )(z , c ) ), ( (x , a )(y ,b )(z , −c ) ) (three secondary tri-
angles).
Note that each edge (x , a )(y ,b ), (x , a )(z , c ), (y ,b )(z , c ) appears in two triangles com-
ing from (x y z ) so each of these edges is a fold.
• Each edge occurs in two triangles: suppose we also have original triangle t = (w x y ).
Then (x , a )(y ,b ) occurs in two triangles from t = (x y z ); (x , −a )(y , −b ) occurs in two
triangles from t = (w x y ) (also a fold) because (x , t ) = − (x , t ) = −a and (y , t ) =
− (y , t ) = −b ; (x , a )(y , −b ) and (x , −a )(y ,b ) each appear in one triangle from t =
(x y z ) and one triangle from t = (w x y ) (so not folds).
• Can follow triangles around each vertex (x , ±1): close up because original degree of
x was even, so have proper rotation.
Example: In K2 + C6 as shown, D has three components with vertex sets {p,q }, {u , w, y }
and {v, x , z }. Assuming all values of t1 are +1, we take t1 + α(q, p ) + α(u , w ) + α(v, x )
which has coefficient 2 for q and coefficient ±1 for everything else.
Note: As mentioned earlier, if all components of D are even order then works for any m ;
Bouchet gives other conditions that will guarantee this.
Folded coverings
If we want to extend Theorem above to even m , will be enough to do it for m = 2, then
can use induction for powers of 2 and combine with result for odd m . But it can be
shown that it is not always possible to get a generative 2-valuation.
Instead, need to use folded coverings [Bo82]. Original coverings have property that two
triangles containing given edge (x , i )(y , j ) correspond to the two distinct triangles
containing x y in G . But for folded covering, may have fold on edge (x , i )(y , j ): both
triangles containing this edge correspond to same original triangle (x y z ).
Theorem: Suppose Ψ is a triangulation of eulerian G . Then there is a triangular embed-
ding of G2) of the same orientability as Ψ, obtained by a folded covering.
Proof: Assign (x , t ) values as previously (±1 values at corners, alternating around each
vertex).
• For each x ∈ V (G ) let (x , −1) and (x , 1) be corresponding vertices in G(2).
• Given a triangle t = (x y z ) in Ψ with a = (x , t ), b = (y , t ), c = (z , t ), replace by four
triangles
( (x , a )(y ,b )(z , c ) ) (primary triangle),
( (x , −a )(y ,b )(z , c ) ), ( (x , a )(y , −b )(z , c ) ), ( (x , a )(y ,b )(z , −c ) ) (three secondary tri-
angles).
Note that each edge (x , a )(y ,b ), (x , a )(z , c ), (y ,b )(z , c ) appears in two triangles com-
ing from (x y z ) so each of these edges is a fold.
• Each edge occurs in two triangles: suppose we also have original triangle t = (w x y ).
Then (x , a )(y ,b ) occurs in two triangles from t = (x y z ); (x , −a )(y , −b ) occurs in two
triangles from t = (w x y ) (also a fold) because (x , t ) = − (x , t ) = −a and (y , t ) =
− (y , t ) = −b ; (x , a )(y , −b ) and (x , −a )(y ,b ) each appear in one triangle from t =
(x y z ) and one triangle from t = (w x y ) (so not folds).
• Can follow triangles around each vertex (x , ±1): close up because original degree of
x was even, so have proper rotation.